David Fairhursta and Stuart Prescottb
aXigo Nanotools Inc., Bethlehem, PA 18015, USA
bBristol University, Bristol BS8 1TS, UK
Introduction
Nuclear magnetic resonance (NMR) spectroscopy is one of the most powerful analytical tools used to probe details of molecular structure and dynamics. It requires very high magnetic fields and, hence, generally uses extremely large, powerful magnets. The advent of small, powerful magnets has allowed much less expensive low resolution NMR instrumentation to be designed, making it feasible to measure commercially important characteristics of dispersion behaviour and performance, including the wetted surface area of particulate suspensions and emulsion droplet size. An important additional practical application is the ability to determine competitive adsorption and/or displacement of polymers and surfactants at interfaces. This article presents a brief overview of these new approaches together with an example of each measurement.
Methods
There are two basic measurements that accrue from dynamic NMR, viz. relaxation and diffusion.
Relaxation
In a relaxation experiment, liquid molecules in a dispersion undergo rapid exchange between the bound state and highly mobile free state. A spin relaxation rate constant, Rn, is determined from the reciprocal of spin relaxation time, Tn. The average rate, Rn(av), is given by:
Rn(av) = ps Rb + pb Rf
where, Rb is the relaxation rate constant for the bound liquid and Rf for the free or bulk liquid [n = 1 for spin–lattice relaxation (T1 method); n = 2 for spin–spin (or spin–echo) relaxation (T2 method)], pb is the fraction of liquid in the bulk phase and ps is the fraction of liquid at the surface.
The most straightforward way to measure T1 is by the Inversion Recovery method;1 however, the measurement is comparatively long compared with the T2 method. In this latter measurement, the Carr–Purcell–Meiboom–Gill (CPMG) method2,3 is typically used. The choice of T1 or T2 is based upon sample characteristics such as suspension solids concentration.
Surface area (SA) measurement by NMR relaxation is based on the observation that bound liquid in contact with a particle surface has a relaxation rate significantly different (often shorter by orders of magnitude) from that of free liquid.
The calculation of SA from the measured relaxation data is surprisingly simple and straightforward:
Rav = ψpS L ρp (Rb – Rf) + Rf
where, ψp is the particle volume to liquid volume ratio, S is the surface area per unit weight, L is the surface layer thickness of fluid and ρp is the bulk particle density.
The layer thickness, L, is assumed invariant with particle size. For an unknown material, it is necessary to obtain an independent estimate of Rb. The other parameters are usually known, or can be measured or calculated. There is no complex deconvolution of an autocorrelation function as in particle size analysis by, for example, dynamic light scattering.
Diffusion
Diffusivity measurements are based on the use of a pulsed-field gradient method.4 An RF field gradient, G, is applied across the sample causing the protons to move. Monitoring the positions with time enables diffusion to be determined; it is the basis of magnetic resonance imaging (MRI).
In an emulsion, mapping out these spin trajectories defines the shape of the droplet because the liquid–liquid interface constrains the maximum random–walk distance that the proton can move within the droplet.
A diffusion coefficient, D, can be obtained by measuring the change in (T2) spin–echo decay as G varies, given by:
I(G)/I(0) = exp [–γ2G2 δ2 (Δ – δ/3) D]
where d is the duration of the field gradient and Δ is the spacing between successive gradient pulses.
For small droplets (<<1 µm), the size can be calculated directly from the diffusion coefficient, D, using the Stokes–Einstein relationship:
D = kbT/6 πηa
where, a is the drop diameter, η is the viscosity of the continuous phase, T is the temperature and kb is the Boltzmann constant.
Although the attenuation function is more complex for large droplets (>1 µm), the dispersed phase cannot diffuse distances greater than the droplet size, resulting in restricted diffusion. This produces an interferogram (similar to scattering experiments) from which the droplet radius can be calculated.
Results
The following are three examples which illustrate the use of NMR relaxation and diffusion as an analytical tool in the characterisation of dispersion behaviour.
Example 1: Determination of the wetted surface area of particulate suspensions
The surface area of particles influences many aspects of product performance such as the hiding power of pigments, the activity of catalysts, food texture and taste, the potency, bioavailability and toxicity of drugs. Traditional surface area measurements, using methods such as gas adsorption, are limited to the analysis of dry powders. However, the overwhelming majority of manufactured products involve dispersions of particles in a liquid.
To illustrate NMR surface area measurement of suspensions, two different active pharmaceutical ingredients (APIs), of approximately the same molecular weight, were used and the results are displayed in Figure 1.

Particle size reduction of any API prior to formulation will significantly increase the specific surface area and, subsequently, the rate of dissolution of the drug (for example, in the gut milieu after oral administration) and may result in significant improvement in the rate and extent of drug absorption so that the bioavailability requirements of the drug are met.5 Any increase in efficacy can reduce the potential toxicity (because less drug substance is needed). There is also a growing body of evidence that, specifically with nanoparticulate materials, it is the surface area and not particle size that is the defining metric that controls toxicological interaction.6
The first API, a synthetic derivative of the naturally occurring steroid hormone progesterone, is prescribed as an appetite enhancer used for AIDS patients and was supplied as a “nanosuspension” in water at 12.5 wt%. The intensity-weighted mean particle size, determined by dynamic light scattering (DLS), was 240 nm with a fairly symmetrical size distribution. The surface area, estimated from this mean size, was 18 m2 g–1.
The average surface area, calculated from the slope of the plot of Rsp vs volume ratio, ψp, is 38 m2 g–1. Rsp is defined as:
Rsp = [Rav/Rb] – 1
The linearity of the response as a function of dilution (viz. initially at full 12.5 wt% concentration and then serially diluted using distilled/deionised H2O down to 3.1%), together with the fact that the intercept value (Rsp at ψp = 0) agrees with an independent value for the bulk relaxation rate, demonstrates the validity of the fast exchange limit.
The second API was a 10% aqueous suspension of a non-nucleoside reverse transcriptase inhibitor, originally developed for use in the treatment of AIDS. The mean volume-weighted particle size of the dispersed material, determined using laser diffraction (LD), was 2.4 µm; the size distribution is bi-modal. The surface area, again estimated from the mean size, was 2 m2 g–1 in comparison with 24 m2 g–1 calculated from relaxation data.
Particle shape, surface irregularities and porosity will inevitably lead to estimated values of surface area from particle sizing measurements that differ significantly from the true value7 and the current results confirm this. In any particle size distribution, the total surface area will be dominated by the smaller sizes, as embodied in the second example in which the smaller fraction (around 0.2 µm) comprises only about 10% (by volume) of the total. Thus, indirect estimate of surface area from particle size measurement can result in misleading, if not erroneous, assumptions about bioavailability etc. Clearly, the directly measured wetted surface area will be the more relevant value.
Example 2: Competitive adsorption
In household and personal care formulations, environmental pressures are driving the need to produce ever more sophisticated detergent blends; new approaches to nanoparticulate API formulations and drug delivery platforms necessitate elaborate stabilisation as they proceed from manufacture to patient; cosmetics and paints increasingly contain mixed suspensions and obtaining optimum stability of each of these multi-component systems poses significant problems.
Hydrosoluble neutral polymers are frequently used in conjunction with surfactants; their adsorption onto particles is key to the preparation of stable homo- and hetero-dispersions. Next, we illustrate using NMR relaxation measurements to study competitive adsorption of such materials onto surfaces.
Poly(vinylpyrrolidone) (PVP) is a water-soluble polymer widely used in cosmetic and pharmaceutical formulations. Figure 2(a) is an NMR relaxation measurement showing the initial strong adsorption of PVP (Mw~55 kD) onto 15 nm silica nanoparticles.8 Sodium dodecyl sulfate (SDS) is an anionic surfactant that does not bind to silica but is known to form a complex with PVP. Figure 2(b) illustrates that, as the SDS concentration increases, the relaxation rate approaches that of bare silica in water, clearly demonstrating the consequent desorption of PVP from the silica surface upon addition of the SDS.

Thus, NMR relaxation measurements can be used not only to study adsorption at interfaces but, potentially, to optimise industrially relevant formulations.
Example 3: Determination of the droplet size of emulsions
Emulsions are used in a wide variety of industrial applications, from precursors for the preparation of polymer microparticles to the clean-up of oil slicks. They are a core technology in cosmetic and personal care products; pharmaceutical uses are found in ocular, topical, mucosal, intramuscular and oral applications. Emulsions offer the formulator an elegant delivery vehicle and can be used to deliver multiple active materials—to keep them separate if they are reactive with one another or if each has a differing solubility, or to present an active material in a format that improves the distribution and/or permeation on application.
Techniques such as laser light scattering are used to measure emulsion droplet size but they require that the system be diluted and the process can induce instability. Thus, there exists a need to measure emulsion droplet size non-invasively.

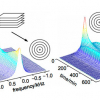
The microfluidic technique can be used to prepare emulsions having uniform droplet sizes from 20 µm to 100 µm. Figure 3(a) is a micrograph of a creamed sample (preparation of which was tedious and time-consuming) of a 10% oil (polydimethyl siloxane)-in-water (PDMS O/W) emulsion which has an average droplet diameter of 40 µm.9 Figure 3(b) shows an NMR interferogram of the PDMS O/W emulsion, measured directly without dilution or any other preparation, from which the droplet radius is calculated as:
Droplet radius = 0.715/the position of the first minimum in Q, where, Q is analogous to the wave vector in scattering theory and in the current example Q is 36,000 m–1.
Thus, the PDMS droplet radius = 0.715/36000 = 19.8 µm
The agreement between the two measurements is excellent. The prime advantage of NMR diffusion to measure droplet size is that it is a simple, fast and non-destructive technique. The emulsion system can be studied without any perturbation that will affect the outcome of the measurements. Further, the system can be characterised repeatedly with no time-consuming sample preparation between the runs.
Conclusions
Low-resolution NMR relaxation and diffusivity measurements can give unique insight into molecular processes and structure. There is high sensitivity to changes in molecular composition and conformation at interfaces. The technique provides unique fundamental insights and so further exploration in both the academic and industrial contexts looks rewarding.
References
- T.C. Farrar and E.D. Becker, Introduction to Pulse and Fourier Transform NMR Methods. Academic Press, New York, USA (1971).
- Y.H. Carr and E.M. Purcell, “Effects of diffusion on free precession in nuclear magnetic resonance experiments”, Phys. Rev. 94, 630 (1954).
- S. Meiboom and D. Gill, “Modified spin–echo method for measuring nuclear relaxation times”, Rev. Sci. Instrum. 29, 688 (1958).
- B.P. Hills, P. Manoj and C. Destruel, “NMR q-space microscopy of concentrated oil-in-water emulsions”, Magn. Reson. Imaging 18, 319–333 (2000).
- R.W. Lee, J. McShane, J.M. Shaw, R.W. Wood and D.B. Shenoy, “Particle size reduction” in Water-Insoluble Drug Formulations, Ed by R. Liu. Interpharm/CRC Press, Boca Raton, Florida, USA (2008).
- K. Van Hoecke, K.A.C. De Schamphelaere, P. Van der Meeren, S. Lucas and C.R. Janssen, “Ecotoxicity of silica nanoparticles to the green alga Pseudokirchneriella subcapitata: Importance of surface area”, Environ. Toxicol. Chem. 27, 1948 (2008).
- T. Allen, Particle Size Measurement, 4th Edition. Chapman & Hall, New York, USA (1990).
- B. Cattoz, PhD Thesis (Bristol, in preparation).
- P. Wiranpootchit, PhD Thesis (Bristol, in preparation).